Discrete Mathematics - Quiz 4
Path Finding
A path finding algorithm is designed to find the shortest path between two points, while avoiding obstacles. It relies on a concept called discretization, which simplifies the problem by limiting the regions of the map into a finite set. To understand how we can move around the map we create relations which describe from where and to where we can move.
Relations
The relation ranging from some set \(A\) to the set \(B\) is a subset of the Cartesian product between the two sets. To denote the relation between two set we use the notation \(a R b\), which can also be expressed with \((a,b) \in R\).
To represent a binary relation in a more legible format other than a list of lists, we can use a matrix.
| R | 1 | 2 | 3 |
| a | 0 | 1 | 1 |
| b | 1 | 1 | 0 |
Binary Relations
The number of binary relations, for some set \(A\) with \(n\) elements, is given by:
\[ 2^{n^2} \]
Reflexive Relations
For some relation \(R\) on \(A\), it has the property of being reflexive if and only if:
\[ \forall a \in A ((a,a) \in R) \]
An easy way to recognize this in a relation, is using a matrix and seeing that all the values alone the diagonal are 1s.
If we want to show that a relation is not reflexive, since it has \(\forall\), we just need to show one case for which it is not true. This is done by finding a pair \(\exists a \in A [(a,a) | a\ \not{R}\ a]\). If this holds true, then we say the relation is irreflexive. This type of relation is defined by:
\[ \forall a \in A ((a,a) \not \in R) \]
If all the mentioned properties of a relation are satisfied, we call it a poset.
Symmetric Relations
A relation is told to be symmetric if it has two pairs which are the inverse of each other. The definition is as follows:
\[ \forall a \in A \forall b \in A (((a,b) \in R) \Leftrightarrow ((b,a) \in R)) \]
To recognize this in a matrix, we need to see if the values are mirrored over the diagonal.
Antisymmetric Relations
For a relation to be antisymmetric, it must be that the non-diagonal values for an inverse relationship have a different value. Mathematically it is defined as follows:
\[ \forall a \in A \forall b in A (((a, b) \in R) \in \neg ((b,a) in R)) \]
For it to be true, there can be no one pair of relations which are symetric.
Transitive Relations
This type of relation is the hardest to detect when looking at a matrix. It is conceptually similar to the hypothetical syllogism. In english, it could be describe as the property of a relation, in which a connection defined by \(n\) relations, can be simplified to just one relation.
\[ \forall a \forall b \forall c (((a,b) \in R) \land ((b,c) \in R) \to ((a,c) \in R)) \]
Relation Composition
The composition of relations, is a relations, which begins in the first term of the first set and ends in the last term of the last set.
\[ \forall a \forall b (((a,b) \in F \circ B) \Leftrightarrow \exists c(((a,c) \in G) \land ((c,b) \in F))) \]
The composite relation of the same set over different variations \(n\) is represented as \(R^n\).
Other Relation Operations
- Converse(Inverse/Transposition)
- This relation, given by \(R^T\) is defined by \(\{(y,x); xRy\}\) where the elements of the ordered pair are flipped.
- Negation
- This operation should give the complement of the original relation set. Represented by \(\bar{R}\).
- Union
- This relation is analogous to binary addition, and can easily be done if we are looking at the relational matrices.
- Intersection
- Similarly to the union, it is easiest to do if we are looking at the matrices, except, this time we multiply the respective values.
Closures
A closure, is a collection of changes which need to be made (only additions) so that a relation satisfies certain property. It must be done with the minimum possible number of pairs.
Reflexive Closure
With this closure, it is our aim to change the relation so that it is reflexive. This is done by adding all the missing connections between \((a,a)\), or simply changing all the values on the diagonal of the relational matrix to 1. If we have to do to do this on a graph, we just add loops to each node.
Irreflexive Closure
This can only be done if there are no \((a,a)\) pair in the relation, because otherwise we would have to remove some and we cannot do that when defining a closure.
Symmetric closure
This closure can be done by adding each missing \((b,a)\) pair for all \((a,b)\) pair. In a matrix, we simply write a 1 for each mirrored value over the diagonal. The easiest way to do this, is to transpose the matrix and add it to the original.
Transitive Closure
The transitive closure of a matrix is done by adding any missing links for paths which can be done in more than 1 steps.
Partial Ordering
A partially ordered set is a set with a relation which is:
- Reflexive
- Antisymmetric
- Transitive
Think kind of set is also referred to as a poset, which is denoted by \((A,R)\), where \(R\) is the relation with all the above mentioned traits.
Hasse Diagram
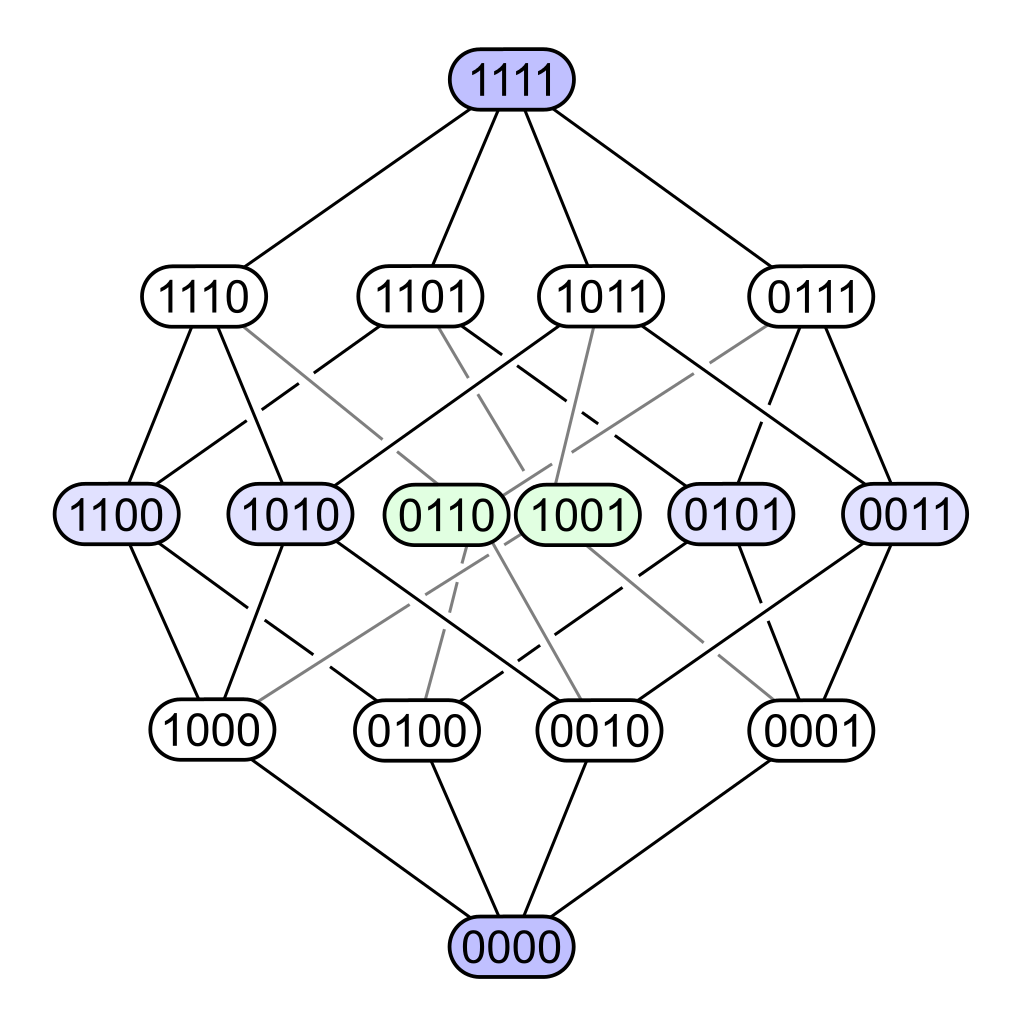
To create a Hasse Diagram from any relation represented by a graph, we have to follow the following rules:
- Make sure all arrows point up
- Remove all loops
- Remove all transitive vectors(connections)